I've had a GregO website in one form or other posted up on the Internet since early 1994 when a good friend allowed me a little bit of space on a university server for my personal use in exchange for some programming services. Since that day I’ve always had a link to some sort of 'Albert Einstein' information web site or tribute site to him posted on my own website. At first it was only a short blurb that I wrote myself but later I linked to more in-depth, professional sites of others that were promoting Einstein's legacy.
A while ago, someone asked me why that was?
I was told that they thought Einstein was boring, that he was just a relic from a past era.
People think it's cool to quote him, but his era and certainly its "world view" wasn't very relatable or honorable anymore.

I paused.
I thought, "yeah?... really?"
So, is he just a meme now?
Has he become just another Facebook/Twitter-posted-caricature, usually used more to suggest the poster's cleverness than the insights and observations that Einstein had?
And I wondered about the 'world view' comment.
It seems that culture now tries to condense and summerize everyone down to a basic category, mindset, or type rather than understand that most people are diverse, complicated, sophisticated tangles of their individual nurture and nature, especially the more refined, gifted, advanced ones among us. I fear that people are forgetting or not learning the value of thinking for themselves and the satisfaction that comes from creating original insights and ideas through labored thought as opposed to the easier, predictable, spoon-fed, flock-mentality style of indoctrination that seems to pervade our lives and institutions right now. And it should be noted that the 'labored thought' thing was quite prominent in the past, now 'unrelatable' eras.
Einstein has intrigued me all my life.
To me he was a perfect example of the deep complexities and surface simplicities that can exist at the same time in some rare human beings and were so prominent and obvious in him. He was an enigma... a deep, mysterious mind yet could see things on a child-like level with profound, unusual clarity.
He can probably be most single-handedly credited for our present society’s concept of ‘the absent-minded professor’. Our image of him as a wild-haired, disheveled older man in deep thought, pipe in hand and oblivious to the world around him was only from how he became largely in his last years, before he passed away. His image has since been the subject of countless cartoons and depictions of a quintessential ‘professor’, even as younger generations might no longer recognize the immediate connection.
Actually, he was always very mentally sharp and quick with a clever quip about any subject. And in fact, while his second wife was still alive, she very much got him to dress more stylishly and would often act as a bridge between him and the social world he was exposed to. She was somewhat of a social butterfly, in the most favorable connotations of that term, and with her, Einstein was often very well dressed and sociable. When she finally left this world, he lamented on how he’d ever be able to cope in that world without her. He missed her a lot and gradually became how he is most generally remembered, alone and preferring the company of equations and mental gymnastics.
Contrary to most lore about him, he was a good student in school and was always perceived as very quick witted and intelligent by anyone who knew him. His biggest problem throughout most of his young life was with authority. He’d often find himself locking horns with teachers or professors because of his outspoken nature and smart-aleck demeanor so they’d give him poor references or be dismissive towards him. Some of this has found its way into history books as disparaging comments about his performance as a student. Einstein, in later life, was aware of this history about him and didn't help the myth much when he once joked, 'When I was a student, I wasn't any Einstein'. Funny guy.
Probably because he could out-think most of those around him and was so incredibly mentally accute, he quickly became bored with their classes or disenchanted with their direction of teaching. Out of frustration he actually researched and taught himself a lot of the contemporary physics that were published in the previous 50 or 60 years but were being ignored altogether by the institutions he was in. The ideas were too new or too controversial for the professors of that time to embrace, most of who were already set in their ways and views. Especially the now recognized work of Maxwell in the area of electromagnetism and accelerated charges.
Einstein was born on March 14, 1879.
Some book I had read as a kid mistakenly said it was March 15th so for years I thought he and I shared a common birthday and, like a kid would, I thought that was kind of cool. Later on I found out it wasn't so. But what really struck me about him was that when I had read a book on the 'theory of special relativity' I found in my town library when I was in grade school, I could understand his concepts without a problem. His ideas were so simple that a child could understand (just as Einstein said they should) yet so profound no one had seen them before. Genius, it seems, is the ability to see things nobody can or from a point of view not previously seen or thought of. It’s the inspired guess born amid the multitudes of crappy ones I suppose. Whatever it is, he had it.
It helped me discover and awaken an inborn instinct that symmetry and beauty lies at the heart of anything true or correct. I believe if you’re confronted with a choice between a solution that involves complicatedly twisting logic or beautiful simplicity, the latter is probably the correct choice even when you’re not sure.
For example, another person who seems able to see the simplicity in things as Einstein could is Paul McCartney, the ex-Beatle. In his world of music I see how so many of his tunes derived from just looking at traditional or common melody lines in a different, fresh way, never done before. The genius of seeing the obvious that the rest usually can’t. His song ‘I Will’, for example, is just a clever twist of a centuries old piano jingle that almost any non-musician knows how to plink out on a piano or at least would recognize instantly.
This clarity of thought is a common theme over and over in the lives of so many great minds in our history. Even a troubled, probably crazy mind like Kepler’s could slide into a place of smooth perfection where the difficult became clearly simple.
E=mc2
Undoubtedly this is the most famous physics equation in the history of the world. We immediately associate it with Einstein and incorrectly with advanced thinking beyond our general capabilities. We’re partially right. It was indeed inspired genius to derive concepts like this the first time, as Einstein did, but now he has, it is actually one of those simple, beautiful little theories that seem to click easily in the mind as I’ve been speaking of.
Just as a little side bar here:
This is an interesting 'rejection letter' that is said to have been found among Einstein's collection of keepsakes and 'official' papers after he passed on.
It's from the University of Bern (letter shown below) . He was applying for a junior position on the staff based on his published paper: 'Special Theory of Relativity' (the subject of this essay). This theory (having appeared in a prominent scientific journal of the time, the Annalen der Physik) had been mostly panned, ignored and unacknowledged by the movers and shakers of the physics community of Europe. Albert was a young man then. He was a 'nobody' in their eyes with no history within the official Physics society and without any formal, impressive credentials. Professor Heinrich, the author of this letter, was an important man in this academic sphere but unfortunately doesn't seem to be a very deep thinker --- based on his decision, and what history has certainly since confirmed Einstein to be.
(BTW I love the 'artistic' reference in this letter...
...says a lot about Einstein and pure 'thinking' in general...)BTW, a further little side bar:
I found this letter on the internet. The thing that bothers me is that it is in English. Einstein's prominent, daily or mother tongue was German, especially at the time of this letter. The University is in Bern (Germany). People in Bern spoke and wrote almost exclusively in German at this time. Yet, this reply is written in English? I wonder about its legitimacy. I suppose it's not impossible it is authentic and that the professor replied in English..., yet I'm not totally convinced.
Maybe it is an 'English' representation of an original 'German' letter? I don't know...
Anyway... regardless of my suspicions...
it is historically true Einstein was rejected for this positon, by this man, at this time...
letter or not...

Anyway... continuing on... speaking of the 'hard work' being done for us...
Suppose you could go back in time with the knowledge of the laws of gravity as they are commonly taught now in high school, or the solution of the carbon filament for the first electric light bulb, or even knowledge of the layout of the continents and oceans of our world. Any one of these would easily qualify you as a bonafide genius in a previous time period and you’d now be in history books. Yes, to understand Einstein’s theorems isn’t too tough now that the hard part is done… thinking them into existence.
You know what part of the problem is?
It’s human nature to develop lifestyles of no greater complexity than is required to carry on in daily life. Part of the reason we all don’t know Einstein’s theory so well already is that it doesn’t appear to be needed in each of our daily lives for us to continue to propagate and thrive. Energy isn’t spent by the populace to delve into it and ensure we all know it well.
Don’t feel bad. This is how we are and have always been. All of mankind.
As an example, it was discovered a while ago that the bushmen of Botswana, for hundreds of years, used a binary counting system. They didn’t use numbers like 1, 2, 3, 4, 5, 6, 7, 8, 9, 10 the way we do (ours being a ‘base-10’ counting system). Instead they used only two numbers to represent different quantities, just like our computers do. One number was pronounced ‘a’ and the other was pronounced ‘oa’ in their language. For example, to represent the quantity of a single item lying on the ground they’d say ‘a’. Two items were ‘oa’ to them. Three items were ‘oa-a’. Four items were ‘oa-oa’, five were ‘oa-oa-oa’. All just combinations of their only two numbers.
But, that is where it stopped for them! They had no number for quantities past 5!
Their binary number system certainly was capable of being expanded further to include more quantities. But for things of quantities of 6 or more in their world, their language simply had a word that meant ‘many’, that’s all.
How come?
Well, and so poetically so, it turns out their lifestyles were so simple and rudimentary that they simply didn’t need anything more accurate than ‘many’ to describe quantities of items beyond 5. They didn’t trade more than an animal or two at a time with others. They usually had smaller families because they were nomadic and travelling was easier in smaller groups. They’d take their one spear, and walk 3 nights, to hunt and kill 2 wild boars to share with their 5 children and one wife and 2 grandparents. They’d stare up at the one moon and not even consider counting the many stars. Their lifestyle and a component of it, their number system, was of no greater complexity than was required to carry on in daily life.
That is probably why we aren’t all talking ‘Einstein concepts’ over coffee and pastry with our friends. In fact as life becomes easier and easier to get through without having to know certain things, they begin to drop away from common knowledge and our society appears to get ‘dumber’ and ‘dumber’. Psychologists call this the ‘dumbing down of society’ but I think it has more to do with interest than anything else.
And that’s all fine, but what scares me is that we may inadvertently ignore something that may indeed turn out to be very interesting to us simply because we didn’t allow ourselves to be exposed to it. And worse still, maybe that person who misses discovering a field of interest may be our next ‘Einstein’ who would have actually excelled in it beyond expectations and the whole world would have benefited from it. As a light-hearted example, imagine Gretzky having ignored hockey and taken up chess instead. Would we be putting little 99 stickers all over our chess sets now instead of wearing replicas of his jersey? Or would Gretzky simply be Wayne Gretzky, normal guy, living out his life in obscurity with the rest of us, a guy who likes to play a game of electronic chess against himself while watching hockey games on TV on Saturday nights?
If you understand quantum mechanics theory (a theory Einstein saw born and growing during his later years but never did fully embrace at the stage it was then), you could certainly argue that this version of Wayne Gretzky has indeed already happened!
The problem with physics that makes it tough for people is that it’s cumulative. It’s not like literature. You can pick up Shelly’s ‘Frankenstein’ and enjoy a good story; good read without ever having read anything like it before or after (assuming you can read of course). Music too is like that. You can hear a piece of classical music, out of the blue, and enjoy all of it even to complex levels without ever having heard anything like it before. But physics is different. You must start from the beginning to understand and appreciate each later stage. You’ll find yourself going over the same subjects again and again but each time rising to a higher level of understanding.
‘So much for your argument of simplicity!’ you say to me.
Listen, physics grew directly out of philosophy from ancient times. In fact if you look at the history of physics and philosophy you’ll see they were directly intertwined most times in the past. Indeed because of this close relationship between the two, we notice that during many years of our western society’s history, science actually moved ‘backwards’ instead of progressing. Remember the old ‘everything revolves around the earth’ theory? It’s a perfect example of this. And until only a century or more ago, physics, as we know it, was indeed a branch of ‘natural philosophy’ in the circles of education. So I think that anything coming from a past of philosophy is bound to bring a slight amount of mental turmoil and mind acrobatics with it.
And the language of physics is math.
Yuck! -you say- Math!
I know what you’re thinking already.
But good math is actually very beautiful. Just as English is the language of Shakespeare’s works, ‘musical notation’ is the language of music, motion and movement is the language of dance or painting can be the language of art. Try to imagine how difficult it would be for me to describe Beethoven’s 5th Symphony to someone who hasn’t ever heard it, using only a paragraph of English words such as this one. Perhaps I could attempt to describe it with a ton of colorful nouns, verbs and adverbs but without the language of musical notation I could never write down exactly how it sounds. If LudwigVan had simply written down how he felt it should be played and sound in his native German language, instead of musical notation on sheet music, it is certain that it would have died with him.
Permit me to use my Gretzky analogy again. When he’s in the midst of a game, amid the action and the play is fast and furious around him, he most likely experiences it all much differently than we, the common public, do. He ‘sees’ and ‘feels’ the game in his mind on a level that allows him to anticipate direction, decide on courses of action and become one with the game. He doesn’t ever think about moving his stick a certain way or the mechanics of skating, he just simply desires a certain action or progression at a particular time and his whole being automatically coordinates completion of it seamlessly. Yet in his past he did indeed learn to skate, learn to handle a puck, learn the rules of the game, watched childhood heros and learned tips and tricks from them, and of course, brought a resource of his own natural talent and skill to the game as well.
This is how physics is.
You discover an interest in it. You start at the beginning and learn about it all. You learn about Ptolemy and how the crazy theory of ‘everything revolves around the Earth’ came to be. You also learn the good stuff like about Galileo, the laws of inertia, formulas on distance, velocity, acceleration from his supposed fun of throwing things from the Tower of Pisa, you learn about the famous but quirky Sir Isaac Newton and his F=MA equation (force=mass times acceleration), that eclipses occur in the same place on Earth every 54 years and 34 days, you learn about doppler shifts, Roemer and his hunch on measuring the speed of light. You thirst for knowledge so you soon learn about guys like Pascal, you learn about the discoveries of thermal dynamics, electricity and electromagnetism, Hubble’s discovery of an expanding universe, Madame Curie, on and on and etc. and etc. Some things are hard to understand, some things aren’t. You find yourself rereading things and looking for alternative sources of information all in an attempt to understand better and more.
This is how physics is.
It grows on you to a point where you don't have to lean on the crutch of the basics anymore and can begin to have fun with experiments and thoughts in your mind, unhindered by the mechanics of it all. You may begin to question and even come up with theories of your own.
Another thing to optimistically consider is that to understand the equations for Einstein’s Special Theory of Relativity you don’t need any greater understanding of algebra (math) than what you get in common high school! (On the other hand, the mathematics of General Relativity, one of Einstein’s other later theories, is a lot tougher. That’s Einstein’s theory where he explains a concept of curved space-time producing ‘a perception’ of gravity by us earthlings. Unless you have understanding of advanced mathematics it's best to just stick to the theory of that one).
Anyway, so far, a lot of wind huh?
Well, it all starts with Einstein’s visualization of a beam of light.
One of Einstein’s most difficult concepts to get your head around is that the speed of light is always the same (approximately 300,000 kilometers per second in a vacuum) no matter who is measuring it. This means, suppose you are standing on the ground, on Earth, and you see a beam of light zip by and you measured it’s speed. It would be travelling at 300,000 km/s. That part is easy to comprehend. Now imagine you were somehow able to ride in a spacecraft through space at, let’s say, 299,000 km/s (over 99.6% the speed of light) and a beam of light zipped by you and you measured it’s speed. Believe it or not, it still would be travelling at 300,000 km/s according to you in your new spacecraft, in spite of your great speed!
This seems contrary to common sense! Surely the light beam wouldn’t seem that fast because you are already moving at almost 99.6% of its speed. You’d think it would only appear to be moving about .4% the speed of light relative to you in your spacecraft, just as another car seems to only creep by you while passing you when you’re both driving down the highway at fast highway speeds. Einstein’s theory explains why this isn’t the case at all and, indeed, this light is moving at 300,000 km/s from your point of view and that in fact, light always travels at its constant speed no matter the point of view, no matter the frame of reference!
What is missing here to have any of this make sense?
Why does it seem like a paradox?
To balance things in our mind a certain aspect of our experience that has been set so rigidly in our thinking must be discarded then remolded with a different perspective. The events of the crazy light speed scenerio seem contrary to expected logic and outcome until we allow ourselves to think about a concept of our existence in a new way. That concept is time and how we believe it works.
This is what Einstein ‘discovered’ at a time (no pun intended) when nobody else had. Even now, just me saying this probably isn’t too shocking at all to you. Even if you don’t yet understand how exactly the concept of time, explained in a different way, will help you to understand the light paradox, I'd wager you're pretty open to alternate possibilities. This is because we are products of our environments. In this modern age we've all been subjected to movies, computers, information, and science fiction books of many sorts. Things of this nature aren't as shocking to our systems as they once were to people.
This certainly wasn’t the case in 1905 when Einstein came up with and published his new theories. In fact, his stuff was virtually altogether ignored by the educated, scientific community of the time for quite a while, let alone the general public.
Years before, old Isaac Newton had postulated that time is exactly the same experience for everyone no matter the frame of reference. He said one minute here on Earth is exactly the same as one minute on Jupiter, as one minute on Halley’s comet flying around the sun, as one minute is riding a beam of light across the galaxy. Newton said there was exactly no difference. So for 250-300 years the scientific community accepted and believed this. Old habits often die hard.
This was just like when the whole world had so much trouble accepting Copernicus and Galileo when they contradicted hundreds and hundreds of years of Ptolemy theory by saying the Earth actually orbited the Sun. In fact, Galileo was actually persecuted for suggesting conventional science was wrong and his theory was right.
To make this all more contemporary, suppose I suggested to you that I could prove right here, right now that a dimension exists right beside you that you can move your hand into and thus pass it through a solid wall. A weird, other dimension we haven’t discovered before or experienced. Suppose I claimed to be able to tell you exactly how it worked and even told you I had mathematical proof of its existence. Your first impulse would be to think of me as a wee bit full of myself, probably loony and maybe for good reason. Now suppose I didn’t work in the academic, scientific world and was a relatively young 26 years old as well? This is the kind of scenario Einstein was waving his theories within. It shows how earnestly he believed in them and the soundness of the proof of his math to put them (and himself) out for scrutiny like that.
It gives us a glimpse into his character, his makeup.
You know what is kind of an amusing anecdote?
It wasn’t until Einstein was directly up against the fact that he had to indeed show mathematical proof of his postulations and theories that he begrudgingly realized that he must embrace math more fully and seriously. He then endeavored to master it completely in order to present proofs of his concepts to fellow physicists. Before that, early on, he didn't really relish mathematics as much as you’d think he naturally would have, being who he was. But, his focus evolved of course and he very quickly became very fluent in the language of mathematics -- though it never was his love. In fact, he’s even been known to jokingly quip that after the mathematicians got hold of his theories, even he didn’t understand them anymore.
He more loved playing his violin, exploring ideas, and philosophizing about things with the ones in his company.
I should also interject here, quickly, that Einstein never did like the term "Theories of Relativity" that his theories had been given by the world. He looked at them from a different point of view. His focus was on the aspects of his theories that always remained constant or invariant, the things that did not change relative to a point of view. Things like the speed of light (also known simply as ‘c’ in physics), the relationship between energy and momentum of a particle, or the strengths of electric and magnetic fields, etc. He preferred and always referred to this work as his ‘Theories of Invariants’.
So this brings us back to our light paradox example way up above. What is said to be invariant or unchanging in that example, no matter the frame of reference? The answer: the speed of light. I have told you that no matter how fast you are moving up to the speed of light, light itself will always appear to speed away from you at the same speed; the speed of light! I also hinted that the reason for this is because our standard perception of time must change or vary. Well, I still mean to get you there.
So what is ‘time’?
Actually, there is no absolute definition of ‘time’ in our world that can be universally applied throughout the universe but we now have atomic clocks that can precisely calculate a measured perception of the event as we experience it on Earth at least.
Since most of us will never leave the Earth in our entire lifetimes, this system seems to serve us well for as much as we'll ever need or experience.
In 1967 the base unit of time on Earth was finally officially agreed upon. The base unit was agreed to be called the 'second' and was objectively determined to be described within the International System of Units (the worldwide accepted scientific standards commonly referred to as the 'SI') as:
(courtesy of SI website)
"The duration of 9,192,631,770 periods of the radiation corresponding to the transition between the two hyperfine levels of the ground state of the Caesium-133 atom."
In normal language this means: a 'second' is the time required for an atom of Caesium to vibrate 9,192,631,770 times (the 'pulsing-shaking' action caused by the releasing of its radiation). This frequency pattern can be observed and captured by sophisticated electronic devices. The pulse is always consistent and undeviating so when a clock's mechanism is constructed around this atomic structure, the resulting timekeeping-device never varies and constantly displays equal and consistent seconds, minutes, hours, days, etc.
We call this invention the 'Atomic Clock'. It is the world standard timepiece and you can easily access it via the Internet nowdays to set your watch to, if you want.
But getting back to the common person's level, there are descriptions of 'time' in mathematical terms that have been developed throughout the years that help us understand and apply the time-concept to our everyday experience on our global world. These are formulas or precepts that help us relate 'time' to a quantity or quality in our minds that we can then think about and use to understand or map the time-experience we live with everyday.
One of the more common ones is:
Time = Distance / Velocity (time = distance divided by velocity)
To arrive at this let's start with something we all know:
We all accept that Velocity = Kilometers (distance) / Hour (time).
As in: I’m travelling at 100 km/h (the posted speed limit).
We see this equation around us everyday while driving around in a car.
We could write this exact same thing in algebra with:
V=D/T
So it also follows, by simply rearranging the equation, that:
D=VT (distance = velocity X time)(more accurately: the change of distance = average velocity X change in time)
And by rearranging it one more time we get:
T=D/V (time = distance divided by velocity)
This is exactly the initial description of time stated above in the first place and is thus proven.
Next:
The speed of light we have already discovered to be constant always and clocked at approximately 300,000 km/s (‘km/s’ is a description of its ‘velocity’ as per the above).
|
If you question things like I do, you may have just had the question pop into your mind as to why I so quickly state that light is indeed a ‘constant speed’. How can I say that? Good question. Saying that the speed of light is the ultimate, constant speed and nothing in our universe can exceed it, is a fundamental assumption made by Einstein. And indeed, being an assumption, nothing says it can't be wrong. However, you must realize that at mankind's current level of understanding of our universe, we haven't yet ever discovered anything that can move faster than light. Absolutely nothing. As far as our best astro-physicists, cosmologists and scientists can tell, the speed of light in a vacuum is the fastest known thing in nature and has so far proven to indeed be a 'constant' through experimentation, observation, calculation and measurements taken. So for now, until some bright, smart person discovers and proves something out there that's faster, it is almost universally accepted as so.
 Here's an interesting image for you: This picture is a scale drawing of the size and distance of the Earth relative to the Moon. The moving yellow beam represents how fast an actual beam of light or radio signal travels as it makes its way from one place to the other. It's thought provoking to see that even at the close distances we tend to think of the Moon and Earth as being apart from each other, it is still far enough away that light takes about a second and a third to get there. For this beam of light to reach the Sun from us it would take just under 8½ minutes... to the closest star it would be around 4.3 years! That means if you had a powerful set of walkie-talkies and you were talking to your new friend on a planet by that far away star, you'd say "Hi, how ya doing?" in your unit. Then you'd have to sit there, on the grass, waiting for 8.6 years before receiving a crackley reply from your new friend, on the speaker ---> "I'm doing well!" |
Ok, with all that said, here we go…
Let’s talk clocks.
A clock is a device designed to carry out a regular, repetitive action over and over and as such can be engineered in such a way as to display the passage of time in arbitrary but even increments on a dial or digital readout for a human being to view. An experience we may have sitting on a rock while we watch the clouds float across our sky can be translated into a ‘period of time’ by our invented clock device. It will tell us, for example, we’ve sat for '120 minutes’ just watching the heavens. It’s a man-made device of course and has been designed to work in close harmony with the actual daily rotation of our specific planet, Earth, around its own axis in what we call a 24 hour period (1 day). It gives us a frame of reference for the 'experience of time' that all our minds perceive as real. Mankind has made this arbitrary time measurement universal on our planet and everyone has similar clocks so we all report the experience of the frame of one ‘minute’ in the same way as any of our fellow human beings do, all around the Earth.
(Are you beginning to see why it is so difficult to actually define ‘time’? We always tend to describe it as the perceived Earthly experience we know it to be or as a concept in relation to devices we've constructed to 'track' it rather than how it actually works or exists (or not) in nature).
With a clock defined I will now use an example commonly used when attempting to explain Einstein’s conception of time.
Since we know light moves at a constant velocity, it would be an ideal candidate to use as a component in creating a clock device wouldn't it? We know that it is so important that one of the features of a clock device is that it is dependably constant and we also know light velocity is very much so. Also, when measuring time, velocity seems to be an intrinsic part of the mathematical ‘time’ equation as we proved in the formula above (T=D/V). The last factor to deal with in that equation is ‘distance’. So let's take care of that aspect by building a device consisting of two exactly parallel mirrors, facing each other on a cart sitting on wheels. An exact distance separates these two mirrors from each other. Let’s call this distance ‘L’. Now let’s say we have a single photon of light (the smallest particle of light) bounce back and forth between these two mirrors over and over, forever, at the speed of light of course (also known simply as ‘c’ in physics).
What we've just done is essentially built a clock device!
One ‘tick’ of time on our new clock will be the time it takes for the photon to travel from one mirror to the other and back again to where it started. In algebra we'd say this same thing this way:
2 times ‘L’ divided by ‘c’
or:
'one tick of time’ = 2L/c (remember: T=D/V? Our clock respects the formula perfectly. Here T=’one tick of time’ D=2L and V=c.)
If we could imagine just for fun that one tick on our hypothetical clock was actually equal to one of our real world ‘seconds’ (even though in reality it would be infinitely smaller) it isn’t hard to imagine that with a bit of additional hardware we could use this clock as an actual measuring device of time here on Earth.
In fact, this is very similar to how real crystal watches work. It was found that certain crystals vibrated at regular and constant frequencies when electricity was sent through them and engineers soon invented ways to ‘capture’ this frequency and manipulate it through circuitry to display uniform, even seconds on a readout in a timepiece.
So back to our light clock.
On the clock cart let’s put a human being. We’ll name him ‘cart person’. He’s on the cart, sitting on a chair beside his clock. Let’s also place another person in our hypothetical world. We’ll call this guy ‘ground observer’. He stands well away from the cart, on the ground, looking at the clock and cart person from a distance.
With the cart stopped, at rest, let’s now fire up the clock and see what our characters see (Fig.1). The cart person, sitting beside his clock on the cart, sees one tick of time just as the observer on the ground sees it. The photon moves directly up to the top mirror, immediately bounces off it and returns directly back down to the bottom mirror again. Both characters experience time to be an equal, common event here.
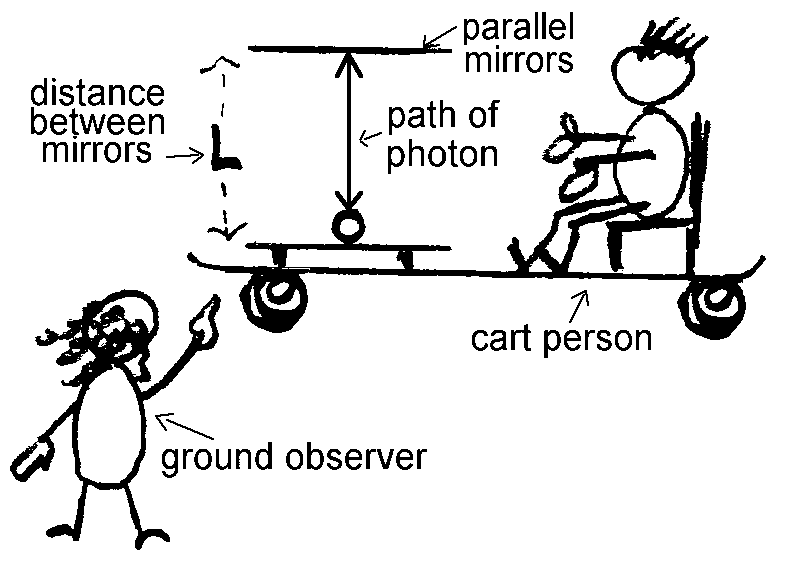
Fig. 1
Mathematically we could put these observations this way, using the formula we just derived a few paragraphs ago:
One time tick for the cart person on the clock cart would be:
tc=2Lc /c (where the ‘t’ stands for ‘one tick of time’ and it’s subscript ‘c’ stands for ‘cart’ or ‘cart point of view’)
One time tick for the ground observer would be:
tg=2Lg/c (where the ‘t’ stands for ‘one tick of time’ and it’s subscript ‘g’ stands for ‘ground’ or ‘ground point of view’)
Other than the frame of reference (signified by the subscripts) the equations are identical and in this case the people in both frames of reference experienced the same passage of time.
So far so good. Nothing new here right?
Now we twist it.
Suppose we now get the clock cart moving at nearly the speed of light past the ground observer (Fig.2).
Something very interesting happens. Because the clock is now in motion (at nearly the speed of light!) relative to the ground observer, the little photon will appear to travel up to the top mirror at an angle and then immediately travel back down towards the bottom mirror at an equal and opposite angle.
We immediately know two things here.
First of all, we know that the speed of light in the universe is constant so this photon is still moving at exactly the same speed for the cart person and the ground observer. Secondly we know that the length of the path it must travel from mirror to mirror has actually increased (as we can see in the picture or measure if we cared to) from the ground observer’s point of view. The photon is no longer travelling the shorter straight up and down path that it did at rest but rather this new, longer angled path both up and down, sloping each way... a zigzag pattern.
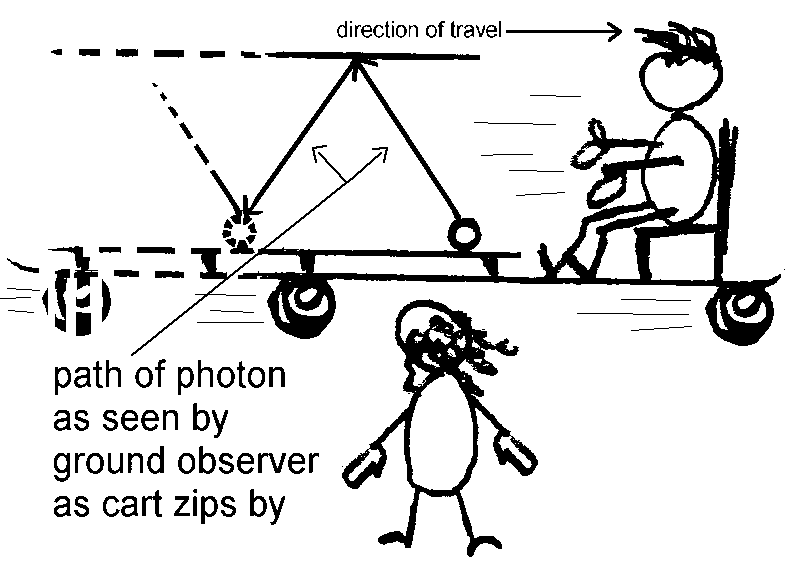
Fig. 2
Mathematically, since T=D/V (time=distance divided by velocity) and we know ‘V’ (velocity of the light photon or ‘speed of light’) is the same in all frames of reference in the universe but the distance traveled by the photon has increased according to the ground observer, we can quickly calculate (using the formula) that time has now decreased on the clock cart from the frame of reference of the ground observer. If the distance traveled by the photon has doubled from the ground observer's point of view, the time would also appear to slow down on the cart to ½ of the ground observer’s time! What initially took the cart person 5 minutes of ground observer time to complete would now take the cart person 10 minutes of ground observer time to complete. So to the ground observer, the cart person has seemed to become a lot slower at completing his tasks. He actually appears to be moving in slow-motion.
Yet another interesting thing happens. The cart person doesn’t perceive a change in time within his point of view of himself. Because he is moving along with it, to him, his clock calculates the same time at this high speed as it did when the cart was stopped, at rest. To him, from his point of view, the photon is moving straight up and down as it always did.
Yet the ground observer did indeed observe a slowing down of the cart person’s time and actions.
It really actually appeared that the cart person was moving in slow motion according to the ground observer.
So conversely, if the cart person looks over at the ground observer at this point, he will see the exact opposite. He sees the ground observer moving around at double the speed that he normally would, in fast motion!
From the ground observer's point of view, time has slowed down on the incredibly fast cart of the cart person. And from the cart person's point of view, time has speeded up for his ground observer buddy. And this is what actually happens!
This brings us to the most interesting scenario of all.
Suppose the ground observer and cart person were both exactly 10 years old when the cart person suddenly accelerated to the before mentioned incredible speed on his clock cart. Suppose also that the cart person lived out his slow-motion life like that for 40 more years of the ground observer’s lifetime. Then let us suppose, after 40 years of the ground observer's time, that the cart person was suddenly able to slow down from his excessive cart speed and return to the exact spot his ground observer buddy was watching him from, during all those years.
Guess what?
The cart person would have actually aged only 20 years during that time away, instead of the ground observer’s 40. The cart person would now be only 30 years old in total while his ground observer buddy would actually be 50! The cart person hasn’t traveled back in time or anything like that, he has just physically aged slower compared to the ground observer because his natural clock had really actually slowed down compared to the ground oberserver's natural clock; meaning time actually slowed for the cart person relative to the ground observer!
The cart person would have experienced only 20 years of his life passing, while traveling at that phenominal speed but he would have noticed everything on Earth speeded up and aging twice as quickly as him if he were able to somehow look over from his cart and watch it all, all those years.
Now, you're probably thinking at this point, that this is all just an interesting little story -- but we can actually prove all these scenarios are in fact a reality in our universe.
More importantly, we can at last begin to discover and realize why a light beam would always be shooting away from us at the speed of light no matter what frame of reference we were to observe it in.
So, to get to the crux of the matter, we only need to invoke a little bit of simple highschool algebra and geometry to describe this entire example and experience, mathematically. Let's continue:
Notice in Fig.2 above that the path of the photon between the two moving mirrors relative to the ground observer forms a triangle with the bottom line being the distance traveled by the cart during the course of one complete tick of our clock. Notice also in Fig. 3 that this triangle can be divided down the middle to form two exactly opposite and equal right triangles. That probably just rang a bell in your mind as to where we’re going with this. In geometry we have a timeless equation called the Pythagorean theorem that is used to solve for unknown quantities in a right triangle. The old a2+b2=c2.
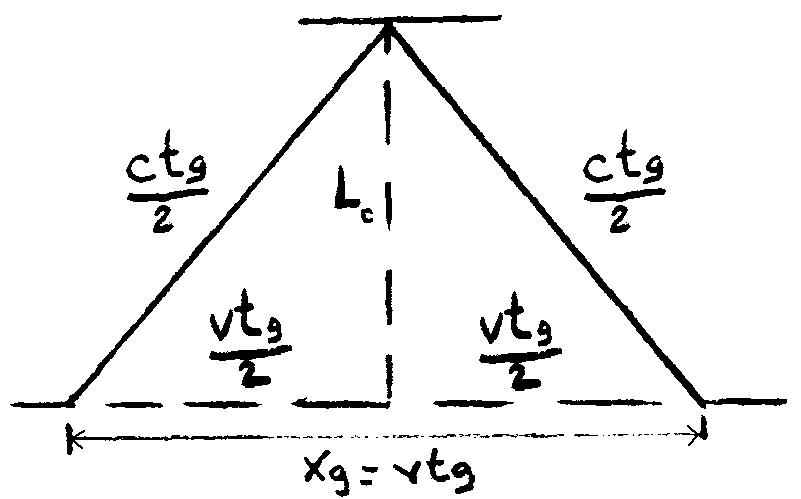
Fig. 3
First of all, the dashed line at the bottom of the big triangle represents the distance traveled by the cart during one cycle of the photon clock (one tick from bottom mirror, to top mirror, back to bottom mirror again). I’ve labeled this distance ‘xg’. I used subscript ‘g’ to point out that this distance traveled is apparent only to the ground observer.
Remember way back, up above, we found that D=VT (distance = velocity X time). This means the entire event of ‘xg’ could also be described as ‘vtg’ or the ‘velocity of the cart during one tick of time as observed by the ground observer'.
Now it follows that if we divide the big triangle into two opposite and equal right triangles those two bottom, equal, shorter sides of each triangle would be exactly ½ the distance each of ‘xg’ and naturally ½ the distance of ‘vtg’ as well. So I labeled each side of the two right triangles appropriately in this way, describing their lengths.
The center, common side to both right triangles remains the dividing line of the big triangle and as it always has, in our examples so far, represents the distance between the two parallel mirrors that the photon is bouncing back and forth between so it remains as ‘Lc’ (the subscript ‘c’ meaning it is the distance from the cart person’s point of view).
We now have distances defined for 2 of the sides of each triangle. Notice that the lengths of both hypotenuses of the right triangles, added together, would equal the entire distance traveled by the light clock in one tick. The photon’s speed is ‘c’ (the speed of light) and the time it took to travel the entire distance up and down the slopes was one ‘tick’ or cycle of the clock (or 'tg’). Once again we know that D=VT (distance = velocity X time) so ‘ctg’ would also represent exactly the entire tick of the clock or as in Fig.3, the entire path of the dark top line of the big triangle. ½ a tick would represent one side only of the big triangle and simultaneously one hypotenuse of any one of the two right triangles. So each hypotenuse is labeled such.
All sides of the right triangles have now been defined and it starts to be clear that the variable in the mix is ‘time’ or ‘tg’ or the velocity of travel of the cart as it whips past the ground observer or ‘v’. In this example ‘Lc’ is a constant or invariant and of course ‘c’ or light speed always is.
It now becomes a simple matter of writing out the algebraic equations to arrive at a final solution.
Using the Pythagorean theorem and our defined sides in Fig. 3 we start with:
(a) c2tg2 / 4 = Lc2 + v2tg2 / 4
By subtracting v2tg2 / 4 from both sides and factoring out tg we get:
(b) tg 2 (c 2 - v2) / 4 = Lc2
or, if we clean it up a bit:
(c) tg 2 = 4Lc2 / (c 2 - v2)
So far so good…
But early on we proved that:
tc=2Lc /c (remember? The ‘time of one tick’ of our photon clock for the cart person?)
By rearranging the equation, this is the same as saying:
Lc = ctc / 2
Squaring this and plugging it into (c) gives us:
Factoring out the c2 from the denominator and taking square roots gives us finally:
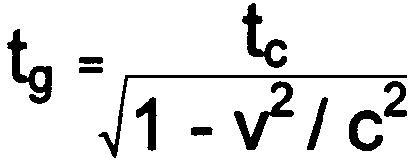
This final solution and equation that we have finally arrived at by mathematically proving our photon clock and light-speed cart scenerio, is famous!
It's the ‘Time Dilation’ equation of Einstein’s relativity theory!
What it proves is that (considering the clock-cart examples we demonstrated above) the time measured by two different people from two different frames of reference is NOT absolute even though it may appear to be equal most of the time in our 'Earth-bound' experience.
It helps us prove that actual time is relative depending on your point of view!
This equation, arrived at by careful examination and consideration of the scenario we set up is just as significant and important to physics as it’s cousin, E=mc2, in my opinion.
So what can we do with this thing now that we've mathematically derived it?
Well, it opens our eyes to some amazing facts about our universe. Let’s look at these:
You can take the formula and plug in some numbers to see what kind of solutions you arrive at.
Allow me to load up the formula for us:
First of all we know the speed of light is 299,792.456 km/s but let’s round it off to 300,000 km/s for ease of calculation. This number would be plugged into ‘c’, in the time-dilation formula. Now, for ‘v’ which is any arbitrary velocity that you can choose, plug in some very slow speed -- something like 100 km/h, the speed of an automobile travelling down the highway. ‘tc’ is a number that will represent any unit of time you choose. Let’s put a ‘1’ here and note that we want it to represent 1 minute.
So what we’ve set up just now is a question.
Suppose I’m in a normal car, driving along, and there’s an observer by the side of the road watching me go by. We both have very expensive, identical watches on that are synchronized perfectly. How many minutes of time will pass for an observer by the side of the highway compared to me, if he was looking at me go by at 100 km/h and we later compared our watches?
After plugging in the values we set up earlier and working out the equation, you’d discover that t g works out to be a value of
1.000000000000000000000000000000000000000. Exactly the same value as t c .
(I was working at a decimal accuracy of 39 places in my calculations to make sure there wasn’t even the slightest variation between the two values).
The time passed for the observer (subscript 'g') and the driver (subscript 'c') end up being exactly equal. 1 minute time for the driver = 1 minute time for the observer. Each person's experience of time was identical when compared to each's observation of the other. This tells us that relativistic time dilation effects are not noticed by us in normal daily Earth life because in everything we do, we move at such incredibly slow speeds compared to the speed of light and the formula proves it!
But now we can have some fun. Start plugging in numbers for ‘v’ (the speed of the vehicle) that get closer and closer to the speed of light and then notice the results of the calculations.
tg (the experience of time for the ground observer) begins to get much larger than tc (the experience of time for the guy in the now incredibly fast car). Time dilates.
Interestingly however, after playing around with the formula for a while, you’ll find that unless ‘v’ is actually a value very close to the speed of light, tg will remain nearly the same as tc.
For example I plugged in a velocity of 299,999.999999999 km/s for 'v', just shy of our ultimate light speed of 300,000 km/s. I found that 1 second of time in my fantastically, ultra-fast car would now equal 12,355,743.3668781 seconds to the observer by the side of the road. His synchronized watch would actually have ticked off that many seconds while mine only ticked off 1 single second. What does a huge pile of seconds like that represent in the real world? 143.01 days. If I could stop my car and return exactly to the spot by the side of the road where I left my observer buddy, I would be 1 second older in real life and he would have aged 143 days in that same time period. Conversely, I would have appeared to be moving in incredible slow motion inside my car, through the window, as he viewed me flying by in my incredibly fast car.
This doesn't hit home as hard until you realize that you can consider that 1 second in the equation to be 1 year instead. Just by changing this point of view, 12,355,743 YEARS would have passed if you stayed away only one year, your time, at that speed and then returned to that spot by the highway! Your observer buddy with the identical fancy watch would have died and gone on to be worm food a long, long, long time by then.
Now just remove a few decimal places and plug in a still incredibly fast, but lesser velocity of 299,999.9 km/s. Let's go back to 'tc' representing 1 second again. The 143 days of the previous example now becomes only 20.41 minutes if this new velocity is used. Significantly less of a difference.
This shows us that the dilation of time only becomes perceptible as the gap between our velocity and that of light becomes very small indeed. You need to be moving pretty close to the speed of light before time begins to become affected in a frame of reference.
This time dilation stuff is very interesting in itself but Einstein discovered another neat thing about his formula.
We can discover odd things happening to the mass of an object that moves closer and closer to light speed.
If you substitute the tg and tc in the equation with ‘m’ and ‘mr’ respectively, where ‘mr’ represents the mass of an object sitting motionless (the subscript r meant to represent 'at rest') and ‘m’ represents the mass of that same object if exposed to velocity, you can calculate what effect tremendous velocity has on the mass of a body.
Plugging in values once again, this time imagine you are pushing an object faster and faster towards the speed of light. After working out various scenarios with different values in the formula you will soon discover that you would require greater and greater force to push the object yet faster and faster towards the speed of light because its mass ‘m’ is continually growing! In fact if ‘v’ = ‘c’, that is, if you could somehow move the object at the speed of light itself, it would become instantly infinitely massive and thus would also require infinite force to move it (remember F=MA). It is impossible for this to happen. It points to one conclusion and indeed a law of nature: No material object can ever travel at or faster than the speed of light, period.
Sorry about your luck Star Trek fans. Your starship moving at the speed of light would suddenly be so unimaginably heavy (infinitely heavy in fact) that there simply isn’t enough matter in the entire universe to generate enough force to budge it even an inch, even a millimeter, even less. It is in fact impossible.
(The science fiction writers of the show get around this setback or inconvenience with their invention of a thing called ‘warp theory’, an imaginary discovery in their future that provides a way to move an object faster than the speed of light. Clever but quite imaginary.)
Finally we come to the last discovery that the formula gives us.
How does high velocity affect spacial reality?
Look back at Fig 1, above. You see my little drawing of a cart with my little guy sitting on it with his photon clock. Now let's take our original time dilation formula but this time substitute Lg for tg and Lc for tc . Lc now represents the actual length of the cart from front to back. Lg represents that same length but how it will appear to an observer on the ground. The formula can now be used to show that the length of an object moving at speeds approaching that of light will change from the frame of reference of the ground observer.
This one is a little bit trickier to comprehend and envision, while working with the formula.
As ‘v’ increases to almost light speed, Lg naturally increases greatly beyond the value of Lc as expected. If we use our last example of 299,999.9 km/s for the velocity, for example and plug in a 1 for Lc to represent a 1 meter cart length, we find that Lg works out to be the number 1,224.74497338848.
The meaning of this result isn't as straight-forward as the time and mass ones.
To understand what is going on here, we have to do a little bit more math with the results.
In doing so we can calculate that Lc represents 0.0816496512929803% of Lg in our solution.
This further calculation now gives us the insight we need to make conclusions of the effect of near light speed on space.
What it means in the real world is that if we could move a 1 meter long object at that speed and observe it from the ground as it zipped by us, it would appear to be approximately .08% of a meter long to us. Along with all the time-dilation and changes-in-mass effects that would occur simultaneously, its length would appear to shrink significantly as well!
The distances for things moving close to or at the speed of light appear to progressively shrink when observed by anyone outside the effect.
(This discovery by Einstein through the use of his formula is significant because this effect was also predicted by a physicist named Lorentz when Einstein was still a young guy. It's now known as the 'Lorentz contraction’ theory and Einstein’s formula inadvertently helped confirm it years later).
So now we can finally return to our question of so much earlier in this text.
We’re riding a super-fast spacecraft nearly at the speed of light and a light ray passes by. The question remains why it doesn’t appear to be moving slower to us since we are almost traveling at the speed of light also? Why can’t we increase our speed so that we could almost reach over and touch it, while it barely moves along beside us?
It’s very simple now, if we've followed the math and reasoning of the time-dilation formula.
It is so very, very important to understand that light speed itself is a mark we can never reach, as we’ve just seen due to impossible infinite forces that would be required to move our infinite masses. You'd never be able to fly alongside a beam of light on your tummy thinking you'd be able to just reach over and grab it as it appeared motionless beside you. You also need to keep in mind that the effects of time-dilation and Lorentz contraction remain neutral or flat on a graph until you are nearly at speeds close to that of light. The line stays pretty much flat over all progressively faster speeds but then shoots upwards very dramatically and exponentially right into infinity, only very very close to and at light speed itself.
As we advance our speed close to within that of light, our actual perceived units of time and units of distance shrink proportionally as compared to what they were when we were motionless on the ground or at lesser speeds. So due to that fact (time dilation and ‘Lorentz contraction’), a light beam will always appear to be moving at exactly the speed of light away from us no matter how close we get to that speed. (Remember V=D/T). Any advances in our speed are only counteracted in exact proportion, at every incremental increase, by the effects of this time dilation and distance contraction.
——
The effect also increases exponentially the faster and faster we get. As we happen to creep closer and closer to the ultimate speed in the universe, the exponential nature of the difference between the bearer of this ultimate speed and our ever increasing one, continues to maintain the vast span of perceptual difference between it and us. Namely, 'c'.
——
This specifically means that we may get one more notch closer to the light beam's ultimate speed but the formula shows us that our perceptions of its time of travel and the distances it needs to cover in that period of time only automatically adjust once again to maintain it's perception of exactly 'light-speed' between us! The ratio is always maintained. If somehow the laws of Lorentz contraction did not exist or occur in our universe and the distances that light had to cover at that incredible speed actually stayed the same in our perception as we slowly crept up to its speed alongside it, then naturally it would also appear to progressively slow down to us. But, in nature this isn't the case, so in any frame of reference we enter, light always speeds away at 'c'.
These changes can be tracked precisely with the time dilation formula at any velocity we choose to place ourselves at, beside the lightbeam!
If you're still confused about these effects of light velocity within space-time, allow me to use another example of how this all would appear in a hypothetical, fantasy world where we all could move at 'close-to' such incredible speeds:
Assume you have two guys, each wearing expensive, perfectly synchronized watches.
Their world doesn't have seconds like ours but a much shorter unit of time called a giggle, let's say.
One guy is in his brand new space-age Cadillac (the Cadillac Guy). The car is exactly 12 feet long.
The other guy (the Garage Guy) is standing inside his fancy garage with a door built into it on each end. This garage is exactly 8 feet long inside when both doors are closed.
Now the Cadillac Guy speeds at nearly the speed of light from a distance, right at the open garage entrance!
What do we see?
There will be exactly two points of view and each will be exactly correct from their frame of reference.
From the Garage Guy's point of view, he will see the Cadillac fly incredibly fast into his garage and fit exactly inside the 8 feet of space of the facilities. Because of the Lorentz Contraction he sees the 12 foot cadillac as only 8 feet long in reality.
He looks into the Cadillac and sees the driver inside motioning in slow motion, yelling with a slowed-down voice like a slowed-down record, to open the back door to let him out. So he yells "No problem!" in a normal voice and runs normally to the back door of his garage and opens it to let the caddy out and on its way again.
Before the car leaves his garage, he also runs to the front to close the open front door right up against the back end of the car.
To the Garage Guy, the car, even though it was measured to be 12 feet long when it was standing still, at that incredible speed will have compressed to 8 feet from his perspective and is, in fact!, only 8 feet long! It fits easily inside his garage with both doors closed but the driver inside the car appeared to move and act in slow motion!
What's more, when the Garage Guy looked at his watch in this fantasy world, he saw the entire length of time the Caddy was actually inside of the garage was a total of 6 giggles of time.
But wait!
There's still the other point of view.
The Cadillac Guy still sees his car as 12 feet long from where he's sitting in the driver's seat!
He goes zipping into the garage at that incredible speed and sees his car's front end touching the closed back door of the garage but to him, the entire interior of the garage appears to have grown to be 12 feet long now from its previous 8 feet and easily holds the length of his entire cadillac!
He waves and yells at the Garage Guy to open the back door and then suddenly hears the Garage Guy yell back "No problem!" in a much speeded up, micky-mouse voice and sees him moving at incredibly high speed to the back door, opening it and letting him out the back!
He's driving out the back end of the garage but notices the Garage Guy (still at unbelievably high speed) run to the front of the garage and shut the front door against the car's back end just as it begins to move away from that newly closed door!
From his point of view he was also entirely within the garage but for less time compared to how the Garage guy saw it. In fact everything outside his Caddy appeared to be move and act very fast compared to his normal speed movements inside his car.
And when the Caddy guy looked at his watch, he noticed the entire length of time his Caddy was actually inside of the garage was only 4 giggles of time compared to the Garage Guy's 6, even though their watches are working perfectly and were indeed synchronized!
Both were exactly correct in their perspectives and both occurred exactly as they appeared!
The experience of time was different for each guy, as well as the appearance of car length and garage length. If we could have somehow weighed the car as it sped through the garage, we would have also found it weighed considerably more at this intense velocity than it had standing still.
We don't experience these sorts of things in our everyday lives simply because we move at incredibly slow rates compared to the speed of light! All of us and our world are sort of like little snails on an Indy racetrack.
Here's another example to help you envision things at near light-speed:
The nearest star to our Earth is 4 light-years away, Alpha Centari. Let's say there was a little planet of aliens circling around it that we wanted to send astronauts over to, for a visit. This means that even travelling at the exact speed of light it would take 4 entire years to get there, from the Earth's point of view watching them fly there and the alien's point of view watching them approach.
4 long years... this seems like a long time to sit in a space ship while traveling to get somewhere, doesn't it?
Surprisingly, it turns out to not be that long at all.
Assuming we did overcome the insuperable engineering problems of creating a craft that could travel any speed we liked, right up to the limit of the speed of light, it would be a very different trip experience for the astronauts. We could actually calculate what the speed of the vehicle needed to be so that the pilots would experience only as much time passage as they desired, before reaching that alien planet so far away.
To us, here on Earth, and to the aliens, the astronaut's space trip will still take over 4 years of time to complete. But to the pilots inside the craft, if they travelled at .99999999 the speed of light, perhaps only days or weeks will have passed for them, in actual time! It could even be just hours (depending on the sophistication of our technology in being able to propel the craft closer and closer to the speed of light).
In fact --- if we really had this space craft capable of approaching light speed, astronauts could theoretically travel the entire Milky Way galaxy (about 100,000 light-years across) in what would be mere minutes or hours or days for them and their ship, simply by adjusting their speed closer and closer to that of light. However, (and it's a GREAT BIG however), from the galaxy's perspective (and the Earth's) 100,000 years of time would have passed when the ship finally came to a complete stop again and everything in the universe would have obviously aged significantly and changed. But for the astronauts, they'd only be minutes or hours or days older than when they left for the trip!
This proves, unfortunately, you can't have your cake and eat it too.
You could zip around the universe, living out your life and having incredible adventures, but nobody (no humans at least) would be around in existence to tell your stories to. The human race would probably be long gone by then and certainly anyone you ever knew would be. In that case, it's probably best to limit your traveling to the stars relatively close to Earth. Then at least you can tell your stories to your great-great-great-great-great-great-great-great-great-grandkids while you're still young.
Yikes! Try to get your mind around that one!
Everything we’ve talked about so far is important and was part of the mental journey Einstein took on his way to derive his most famous formula, E=mc2. We've just seen how his initial questions about light led to his discoveries of the previously unknown intimate relationship between space and time. That in itself would be more than enough accomplishment for any physicist's lifetime career, but he didn't stop there. He went on even further with observations and questions about light that eventually evolved and led to his discovering and proving an intimate relationship between matter and energy as well!
So before we jump right to the matter/energy theory, we need to discuss one more step Einstein took along the way to it...
As I’ve tried to show, it all started with a puzzle he had in his mind, dreamed up when he was still a teenager, of traveling beside a light beam and how it would appear in that environment if you could. (I took a bit of poetic license in my examples using a spacecraft. Einstein liked to use trains in a lot of his examples. We’re all children of our times). This led to his deriving the famous ‘Time Dilation’ equation showing us that the speed of light could indeed be a constant in any frame of reference because space-time itself changes. The invariant of lightspeed and its affected variants of space and time are intimately entwined throughout the universe.
But during this time, all around Einstein, other sharp people were experimenting and postulating as well. With the growing popularity of electricity, scientists had been playing around with sealed glass tubes with gasses injected and sealed inside of them and with electrodes penetrating the glass walls. Electricity would be hooked up to these electrodes and it was found the tubes of glass and gas would light up and glow.
(One practical invention that evolved from this experimentation was the neon light, used on the front of almost every Blues/Jazz club in New Orleans and casino on the Vegas strip. Another was sodium lamps that can light up an entire factory floor or farmyard. And the florescent tubes that light our kitchens).
But let’s take a bit of a side-road here because I think it’s important that we clarify the definition of ‘light’ a bit right now:
We commonly think of light as that thing that we see everyday with our eyes. This is what is known as the ‘visible light spectrum’ but actually visible light is only a tiny segment within a much greater electromagnetic spectrum of radiation. Visible light would more properly be called the ‘visible radiation spectrum’.
Why?
Let me start with an analogy using the concept of sound. We all know that elephants are known to be able to hear and generate sounds that humans simply can’t. Very low sounds. We also know that bats are able to hear sounds far higher than any we can.Bats hear and shriek higher what? Elephants hear lower what?
The answer: frequencies.When you strike the key on the piano for ‘middle-C’ the mechanism hits the corresponding strings causing them to vibrate and this vibration pushes the surrounding air and creates invisible waves in our atmosphere that radiate outwards from there. They travel to your ear causing your eardrum to vibrate in kind. Your brain interprets the signals transmitted to it by your eardrum, vibrating bones, all the way down to corresponding neurons and you perceive in your mind a note of sound in a certain range.
The vibration of the string determines its range. Vibration is the moving back and forth or cycling of a thing. How often this repetitive action happens is called its ‘frequency of occurrence’ or simply frequency, for short.
If you take a single string on the piano and increase its tension by tightening it, after you strike it, it will vibrate even faster and you will perceive this increased frequency as a higher note.
Eventually, if we keep increasing the frequency of the sound, our brains will no longer be able to ‘hear’ it. We simply aren’t built to be able to perceive it. But bats still can. For reasons suited to their evolvement, their ears and brains can perceive frequencies of sound far beyond our own. Dogs apparently can as well. If you’ve ever blown a ‘silent’ dog whistle and watched as the dogs of your neighborhood looked your way, freaked out and started barking you’d realize this.
Light operates in much the same way. Red light appears red to us because our human brains, through the mechanism of our eyes, perceive the frequency of radiation in this range as red light. If the frequency of the light radiation is increased, our brains perceive it as yellow. Increase it some more and we perceive it as something we call green. In fact normal humans are able to differentiate between almost 5 million subtly different frequencies of visible light (or colors).
Increase the light frequency beyond what our eyes can see or brains can perceive and suddenly it will appear invisible to us but its still there. It crosses into a range we’ve labeled ‘ultra-violet’ (that’s just beyond our visible violet color range). Here the frequency of the radiation becomes energetic enough to affect our skin as noticed if you stay in the sun too long or bake yourself under an ultra-violet radiation producing ‘suntanning’ lamp.
And onwards it goes. As you increase the frequency of the radiation far beyond visible light it becomes more and more energetic. X-rays. Gamma waves.
My point in all of this is that visible light is simply radiation of a particular frequency. So from now on, when we refer to light, remember we are including all electromagnetic radiation frequencies because they are all part of exactly the same family. Light, radiation. The two terms can usually be used interchangeably and you might notice that I do from here on, in this text.
Sorry for the sidebar here -- I just didn’t want you to become confused as we started discussing light or radiation beyond our visual range. I hope you now see it is really all the same thing.
Let’s return to our story…
More and more experimentation with glass tubes and electricity revealed that if you put certain fragments of metal inside the tube and bombarded it with electricity and energized gasses, other tubes containing certain gases that happened to be sitting idly nearby on the laboratory benches, not hooked up to anything at all, would sometimes light up and glow on their own. It was soon discovered that they were picking up energy radiating invisibly from the electrified tube somehow and the gases inside these isolated tubes were being stimulated just as if they had been hooked up to electricity themselves! (This isn’t far from how X-rays were discovered actually).
What invisible thing was traveling through the air causing this to happen?
It was all very interesting and puzzling to physicists and scientists.
In spite of being unable to explain the exact causes of this phenomenon, discoveries and advances in electrical technology continued and yielded devices by inventors that enabled experimenters to control the frequency of a light source. This was new. These devices became more and more refined until scientists soon had gadgets that could create radiation (light) in the ultra-violet and x-ray range at will.
Around the turn of the century, a famous experiment was conducted using an experimental setup. For the sake of simplicity, it can be described as a sort of capacitor connected to a battery so that an electric field could be generated between two small plates of zinc metal and the electron current measured on each side of these. One of these small sheets of zinc metal was exposed to a strong light source (a visible light source). The light was shone on it and gradually increased in brightness. (Making a light source even brighter is simply increasing its amplitude. To visualize amplitude, think of it as the height of an ocean wave. We could say the higher the wave, the higher its amplitude). Using the technology of the day, it was discovered that no matter how strong the light got or more specifically, how much you increased the amplitude, no energy was detected to be emitting from the sheet of zinc when the voltage was lowered below a certain threshold.
Then, using their newly invented devices, the experimenters were able to increase the frequency of the light (radiation) source instead of its amplitude. (Visualize frequency as the number of waves moving across a set distance in the ocean, regardless of their amplitude). They found that as the radiation frequency crossed from visible light over into the ultra-violet range the zinc sheet suddenly ‘came to life’ and began emitting energy (electron particles) across to the other sheet of this capacitor device despite the voltage being held at the level that previously had shown inactivity.
Because the energy emitted from the zinc could only be detected if the frequency of the radiation source was raised to and beyond a certain point, in the ultra-violet spectrum, Einstein realized that something was being stimulated enough in the radiation to knock individual electrons out of the zinc. This same thing in the radiation was unable to do so before it reached this certain frequency.
What could this invisible thing be, he wondered?
If something can produce a force strong enough to impact another thing, it must have energy.
Particles can have energy.
He suddenly realized and concluded that light (radiation) itself was indeed displaying the properties of a particle!
You must understand that this was a new, novel concept at this particular time in history.
By this time, in the scientific world, it was widely accepted that light seemed to travel in waves only. One famous experiment, first performed by a man named Young over a hundred years earlier, using a light source and two slits cut in a card that the light was directed through to a flat panel behind, seemed to confirm this wave theory of the properties of light. I won’t go into the details of this famous experiment in this text but only say that it helped establish the wave properties of light as a fact for a lot of the scientists and physicists of that time and even most of them now.
(One of the rules of science is that confirmation through experimentation and accurate data collection supercedes theory almost every time. My only caution to others on this point would be to realize that almost any observation can be rationalized in a certain way to support a theory, but that's a whole other story (you've probably noticed I have difficulties in accepting some concepts)).
Einstein’s concept was significant at this point. No one had ever suggested that light could have properties of a particle before this. Physicists were stuck on the ‘wave’ definition of light. But Einstein did not say light didn’t have wave properties (because it had already been shown by experiment that it in fact seemed to), he only said it ALSO has particle properties.
Now what I really stress here are the words particle properties. Photons do not have mass. But they indeed have energy (E=mc2 proves that the two are the same thing in different forms). That is why it is always said that light can behave in a way that it appears to have properties of particles rather than saying it is made up of particles. Particles, to me, have mass. Photons don’t, they are pure energy. Even if they could have mass at some unimaginable minute quantum level, it would be incalculable.
Remember way back, up above, when we proved that anything with mass in the universe that could hit the speed of light would immediately have infinite mass and thus would require infinite force to be moved and that was impossible to achieve. Light obviously moves at the speed of light so it follows that it can’t have mass. Even something that weighs as little as a single grain of table salt couldn’t move at the speed of light because it would weigh ‘infinity’ by then and couldn’t be pushed by anything in existence.
Einstein took the revolutionary step and posited that light (radiation) indeed at times acts LIKE a thing composed of particles instead of only as a wave. He called these things ‘quanta’. He said that each ‘quanta’ carries energy (called ‘hv’ in physics). Think of them as tiny packets of pure energy. He suggested that because an element like zinc only seemed to eject its energy after being bombarded with a certain frequency of light (radiation), it clearly pointed to the existence of particle-like packets of energy hitting electrons and knocking them about sort of like colliding pool table balls.
He mulled over the concept and derived the simple formula:
Eelectron = hvlight - Ethreshold
‘Eelectron’ is the energy produced by the event
‘hv’ is the energy of the little ‘quanta’ packet (‘particle’ for want of a better word) of light
‘Ethreshold’ is the amount of energy that needs to be risen above to knock an electron out of a given metal.
This equation shows that if the energy of the light packet is below the threshold required to whack an electron of that particular metal out of the orbit of its molecule, nothing will happen. This is seen in real life if we stick a sheet of zinc out in the bright sunlight on a summer day. Nothing happens except the metal getting a little bit warm from the sun. But stick the zinc in the vacinity of an X-ray source, thereby raising the frequency of light (radiation) striking the zinc metal, and electrons begin to leave the zinc. This is because the much higher frequency of the radiation packets produced by the X-ray device is kicking electrons out of the metal, big time! ‘hv’ (the energy of the ‘particle’ of radiation) has increased beyond the threshold required to break the electrons of the metal free.
Over 20 years later, Einstein’s ‘quanta’ became known as the ‘photon’ and the name has stuck. It was eventually accepted and embraced by the scientific community. It is actually his work in the area of photons and predicting how they would act around hugely massive objects that eventually earned him a Nobel Prize and not his Special Theory of Relativity. These days, almost any ‘joe-blow’ physicist discovering yet another microscopic particle in an atom accelerator/smasher experiment seems to get a Nobel Prize for it but something that changed the course of physics like Einstein relativity work didn’t. Funny huh?
I’ve often thought that because Einstein’s formula helped develop the most powerful bomb device the world has known and Alfred Nobel himself invented dynamite, surely there’s a kindred spirit in there somewhere? Hehe
Anyway, some time after he had released all his work on this photon stuff in 1905, he postulated that the momentum (known as ‘p’ in physics) of a photon is:
p = hv / c (The momentum of a photon is equal to its energy divided by its speed (which is always the speed of light ‘c’)).
Why did he work out this formula?
Well, because the classic definition of momentum is mass times velocity but since photons are pure energy and have no mass Einstein realized he had to discover a new way to describe their momentum given that this massless momentum of a photon was obviously able to knock real electrons out of metals. And so he did, but the math is a little bit too complicated to get into in this text so I only show the results of his work and hope you are OK with that. (A hint to some of his logic to derive it is in remembering that we now know that energy and matter are directly related and interchangable).
Also, knowing that ‘hv’ is equal to the energy of the photon we can immediately derive from the above:
E = pc (for photons)
The energy of a photon is a product of its momentum and its speed (which is always ‘c’). It seems matter-of-fact/logical to see the end formula like this but the background mathematics that prove it are very involved and complicated.
This equation is very important in its own right but I’ll also be using it to help us present a reasonably simple derivation of E=mc2.
So why did I just spend all this time talking about this chunk of photon stuff?
Well, because it was an unknown solution to an issue at the turn of the century when experiments were displaying unexpected and strange results that scientists couldn’t prove or explain especially since they were all stuck in mindsets of light 'wave theory'.
But even more curiously and certainly more importantly, if you examine this photon stuff more closely for a little bit, it also clearly suggests a relationship of a certain quantity of energy being able to displace a certain quantity of matter! This is the whole background premise of the Special Theory of Relativity and I'm sure these previously undiscovered relationships between matter and energy instantly clicked with Einstein as well, as he pursued a way to prove that relationship to the world.
So now we finally come to it. The big kahuna —— the 'mc2' thing!
Let’s do this thing…
In nature there is the existence of a conserved quantity called momentum. Most people know it better by what it’s commonly referred to as: ‘inertia’. It is a law of nature and of physics. It’s basically governed by the rule that things that have started to move tend to continue to move in a straight line unless acted upon by some force and things that are just standing still tend to stay standing still. The law called the ‘conservation of momentum’ also dictates that the total momentum in an isolated system is always constant. You can get out of it only what you put in, or have in, in the first place. You can never get more momentum out of an isolated system than it had to begin with.
So much for perpetual machines and people that say they're possible. That's another myth busted.
Hey!
But what about a bomb?
A person could argue that before a bomb explodes it has absolutely no momentum but after it does explode its pieces have considerable momentum each. Isn’t this a violation of the law of ‘conservation of momentum’?
No it isn’t.
Let me explain why.
It’s funny sometimes that we human beings, having evolved and grown up in our little environment of the planet Earth during our entire existence, sometimes don't realize or recognize that things happen around us in a particular way because the laws of nature ensure they happen that way.
I’ll bet that hardly anyone actually wonders why, when a bomb explodes, the pieces of it fly off in seemingly random directions and not in a single direction. It’s exactly due to nature’s law of ‘conservation of momentum’!
The bomb’s initial momentum is 0. The law states that it must also be 0 after the bomb explodes. Momentum is described in the language of math as p=mv (momentum is the product of mass and velocity). Velocity is distance over time (remember 100 km/h?). So the only way the momentum can be zero afterwards is if, for every fragment flying off in one direction, there are pieces flying off in opposite directions. The nonzero momentum carried by one fragment would simply be canceled out by a nonzero momentum of a fragment in an opposite direction.
If we did the math it would be like: -1 +1 = 0.
In a highly chaotic situation of an exploding bomb, in reality it would be very, very difficult, if not impossible, to make all the calculations of every single minute and not so minute fragment of the explosion. But the laws of nature assure us that each and every time, the momentum calculation at the end, if we could somehow measure the mass and velocity of every single part of that exploding bomb, would indeed equal 0, just as it was in the beginning of the event.
It has to be. If this were not true, it would mean we are creating energy from nothing at all and that is impossible.
So it is important for me to touch briefly on the topic of momentum like I have and for you to digest this rule about it.
OK, to derive E=mc2 I want to start off by talking about seesaws:
Assume we have a simple seesaw (is there any other kind?) composed of a long plank sitting perfectly centered on a fulcrum or center point. We also have a couple fat boys, identical twins actually, dressed, looking and weighing exactly the same in every way. At exactly the same distance from their end of the plank each boy takes a seat, sitting perfectly straight and still, opposite of each other. (Fig. 4)
Fig. 4
m = mass of the object or boy
x1 or 2 = the distance of each mass from the center of mass or balancing point
xcm = the center of mass or balancing point
Experience and common sense tells us that the seesaw will be perfectly balanced and as such the plank will be exactly level and parallel with the floor and the balancing point or ‘center of mass’ of the system will be exactly at the halfway point of the plank.
And this is exactly what happens. Of course what is going on here can be shown in the language of physics, your buddy and mine, math! (I know, I know… I can hear the groans).
I can lay down the following equation to calculate exactly where the center of mass on the plank will be:
(a) xcm = (m1x1 + m2x2) / (m1 + m2)
This is easy to see but if we assume the mass (weight) at each end of the seesaw is equal as we do in our example then my equation is unnecessarily bulky. Our two masses being equal can be simply labeled ‘m’. If I make this change then the equation can be simplified as such:
(b) xcm = m ( x1 + x2 ) / 2m
Factor out the ‘m’ and I get:
(c) xcm = ( x1 + x2 ) / 2
This makes it even easier for us to see that the balancing point between two equal masses is the point halfway between them. Common sense. Indeed if we made xcm equal to the value of 0 then x1 = -x2 which is just another way of saying that the masses are located at equal distances from the center of mass or balancing point. One boy is sitting 5 feet from the center and the other is sitting -5 feet from the center. Same difference.
Ok, let’s dig in a bit deeper now…
Remember way back that we saw that velocity is the rate of change of distance (position) with time? V=D/T. If I wanted to apply the concept of velocity (to thereby also introduce an aspect of time) to the center of mass of our seesaw I’d have to adjust equation (a) to this:
(d) vcm = (m1v1 + m2v2) / (m1 + m2)
The equation can now describe the velocity of our center of mass if it became a moving system, or more specifically, if we were to change the masses of the two objects at each end of the seesaw and make them unequal in some quantity. Naturally this would shift the center of mass or balancing point of the seesaw and my new equation (d) could calculate the change of position of this point on the plank over a period of time needed to complete that change.
However…
Notice that the new terms in the numerator are actually just representing our friend ‘momentum’ (p=mv). Because of this, the numerator of this equation represents the total momentum of the system. But remember we just learnt that the law of ‘conservation of momentum’ dictates that the momentum of an isolated system is always constant. This specifically means, most importantly, that the velocity of the center of mass of an isolated system cannot change! If the total momentum of the system is initially 0, then our equation proves that vcm is also 0 and must remain 0! This means the center of mass cannot ever change position! This is a significant concept. (Remember very early on when I said learning physics is a process of reading things over and over again and by doing so you gradually rise to higher levels of understanding? This is one of those times * grin *).
Let’s now modify our seesaw system a bit. Let’s pretend the seesaw itself is now massless (this keeps irrelevant, unnecessary data out of our calculations). Let us also imagine that we have securely bolted to it two equal masses (‘m’) at equal distances from the center of mass. We’ll say the center of mass = 0 and the mass on the left is located at ‘-x’ and the mass on the right is located at ‘+x’ (Fig. 5).
Fig. 5
Using the previous equations, either (a), (b) or (c), we discover that indeed:
m(-x) + m(+x) = 0
Now we twist it!
Suppose the mass on the right side of the balanced seesaw suddenly throws off a burst of radiation directly towards the left side mass. We recall our high school physics that taught us Newton’s law that states ‘for every action there is an equal and opposite reaction’. Since we’ve established our seesaw (that our two equal masses are bolted to) is a massless apparatus, the entire system will recoil to the right on the fulcrum because of the radiation burst (Fig. 6).

Fig. 6
The radiation will travel at ‘c’ (all radiation travels at the speed of light because light and radiation are the same) towards the left-hand side of the seesaw and eventually hit the left side mass and halt the motion of the entire system, once again according to Newton’s law. Before it is stopped, however, the system will have moved a distance of ‘ ∆x’ (∆ in algebra simply means ‘change’ or ‘a change in’) to the right. And finally let us also assume that during the burst of radiation from the right mass, that object lost some of its mass ( ∆m) and the mass on the left gained some mass when the radiation later crashed into it (∆m).
Fig. 7
But now we have to remember what we discussed just a few paragraphs above under equation (d). The law of ‘conservation of momentum’ rules that the center of mass of the system cannot have moved! So after our seesaw stops moving once the radiation crashes into the left side mass we must describe the state of things as:
(e) 0 = (m + ∆m) (-x + ∆x) + (m - ∆m) (x + ∆x) (notice everything must equal 0 because of the law!)
If we multiply this all out and cancel out the appropriate terms, we end up with an equation describing a mass loss:
(f) ∆m = (∆x) m / x
What happened to the mass?
At this point our equation has reached a stage that is still too incomplete to explain this but we can work the equation even further and see where it takes us.
We can compute ∆x.
The distance the masses traveled on the massless seesaw is just ‘vt’ (remember way back earlier? D=VT, a change in distance = average velocity multiplied by average time). ‘v’ represents the velocity of our seesaw when it was kicked to the right in response to the radiation burst and ‘t’ is its travel time doing so.
Let’s tackle time first.
How long was our seesaw in motion? Remember that we said the distance of the right mass to the ‘center of mass’ was ‘+x’ or simply ‘x’ (same thing). We also said that initially, the two masses were located at exactly equal but opposite distances from the center of mass so naturally the entire seesaw length is ‘ 2x ’. The velocity of the radiation which we’ve learnt is just a higher frequency of light is, of course, the speed of light or ‘c’. So now knowing all this, we can calculate the time it took for the burst of radiation to cross the seesaw to the other side:
T=2x/c (remember our T=D/V equation from way back that is used to figure out time?)
By the way, for the sake of simplicity, I’m not counting the distance the seesaw moved while the light was in transit. We’ve just calculated the time for our burst in the previous equation so plugging this into the D=VT equation we get:
∆x = 2vx / c
Plugging this calculated value for ∆x into (f) now updates the equation to:
(g) ∆m = 2vm / c (I’ve already factored it out and cancelled the redundancies) .
Finally, let’s compute ‘v’ (the velocity) in (g).
The burst of radiation from the ride-side mass obviously carried with it a certain momentum (p) to the left. Once again, by the law of ‘conservation of momentum’ our seesaw had to have moved to the right with the same momentum. (Every action has an equal and opposite reaction). Because we’ve stated our seesaw apparatus is massless the total mass of our seesaw system is just the combined masses of the two objects bolted on each end of it or ‘ 2m ’. (We’re not counting the mass carried by the burst of radiation here for the sake of simplicity). So the momentum of the seesaw as it moved to the right had to have been p=2mv (the combined masses of the two objects times a velocity). If we now substitute the identical value in (g) for our just calculated momentum we get:
(h) ∆m = p / c
Now remember at the beginning of this section how we talked about Einstein’s work with photons and their momentum where he found that the energy inherent in a pulse of light or radiation (photon) is just E=pc ? This equation can also be rearranged as: p=E/c.
If we plug this into (h) it becomes:
(i) ∆m = E / c2
This equation tells us that the burst of energy given off by the radiation has a mass ( ∆m ) associated with it.
This answers our question of where the missing mass disappeared to. It was converted to energy.
If we call the amount of mass exchanged in any process where energy is given off ‘m’ then we can write this into equation (i), rearrange it a bit and come up with:
(j) E = mc2
Now that looks familiar, doesn't it?
The reason this formula can be derived is that ‘conservation of momentum’, as I talked about briefly earlier, is extended to include energy as well as mass which causes us to realize that energy is simply a variation of matter.
In deriving it here I made a couple approximations, as you may have noticed and I pointed out, to speed things along but doing the whole thing properly, in detail, as Einstein did in his proofs, yields the same answer.
You know, most people think (and I too made a little joke of it earlier) that Einstein is the reason we have an atomic bomb. To the contrary, his formula doesn't tell us how to get the energy out of matter much less if it is indeed even possible to do so at all. It merely states that there is a real association between energy and matter, that's all.
Once other scientists and engineers discovered a way to build a device that could tap into the energy stored in matter, it turns out that, even up to present day, it is an extremely crude method as far as efficiencies are concerned. In nuclear fusion and normal chemical reactions that can produce energy from matter most of the matter never gets converted. Normal chemical reactions, for example, release less than a millionth of 'mc2'. A hydrogen bomb releases less than 1% of 'mc2'. It's kind of like a person buying a great big bag of potato chips to eat for nourishment and taking the tiniest of nibbles from the very edge of one chip then simply putting it back in the bag and throwing the rest away. The only saving grace in all of it for the scientists so far is that the tiny nibble sure packs a punch!
We still have a long way to go before we eventually discover how to harness this energy in a reasonably efficient way. I'm reminded of that scene in the movie 'Back to the Future' when the Doc needs fuel and starts grabbing banana peels, egg shells and aluminum cans from the trash to throw into his little nuclear fusion device on his car. I'm afraid that future for us is a very long way off yet.
So that’s about it reader.
I’ve studied these equations so many times over the years that it all appears pretty straightforward to me but at first glance you may not agree. If not, I hope that after reading it through a few times it will all start to make sense to you or maybe even inspire you to seek out more information on it all from other sources. At very least it would be nice if you come away with something you possibly didn’t know before and it piqued your interest a bit.
If not, well, maybe my buddy was right, maybe Albert IS boring after all?
Opinions, too, are relative after all…
P.S.
I have a side thought while I'm on the subject, then I'll shut up:"Theory" in common 'street' parlance means a guess, a conjecture, a supposition. It isn't generally considered as a very serious statement but maybe only a loose notion of something that someone believes; a person's particular point-of-view. But in Science this is not the case. In Science, "theory" commands so much more. In science it is the pinnacle of conclusions, it is the -- ultimate -- conclusion after a long, painstaking process of proving a hypothesis. It is supported by proofs that are supported by reasoned, logical, detailed explanations, processes, and sound, empirical evidence.
But even after having said that, I want to offer a small caution. I would suggest that a scientific theory, even presented with its ominous gravitas, still needs to be approached cautiously and tentatively as well. Even though 'theories' are considered the optimum of the scientific realm, the crown of the research, you must be aware that a theory is just some sharp person's best guess so far; albeit backed up with sound evidence and tested by the scientific method. It is the "state-of-the-art" position on a particular subject, situation or occurence, at this time. However, it's possible it could change. It is possible it may be proven wrong or flawed at some time in the future if further evidence or knowledge arose; it simply represents a current optimal explanation of our reality as we perceive it at this time based on our best science and evidence to date. And that's good.
I would also caution that you understand that results of any experiment or observation can sometimes be twisted to conform with a biased point of view of the investigator and even the mathematics may be nudged to agree with the position of the person(s) conducting the experiment. Hopefully this doesn't happen often and the science is pure and the people honorable.
This is why the scientific method demands that the process be able to be peer reviewed; that methods, experiments and processes be able to be duplicated by other parties, and that claims are falsifiable. Yet I can't deny the uncertainty of human nature and the forces of self-gratification and the seeking of personal fame or acknowledgement that exist. But probably the greatest corrupting force would be the basic financial or economic dependencies researchers develop and incur while conducting their research, (the burdens of raising their kids, paying mortgages, buying groceries, pleasing their wives/husbands, upkeeping the car, etc.) all the while, cultivating their personal professions. There is tremendous subtle social coercion to tow-the-line-of-thinking of the sponsors, patrons, and cohorts. This, I believe, is the largest pressure on most persons involved in scientific research these days --->; even up to very large associations of scientists, university professors, researchers (the 'global warming' debacle shows how loosy-goosy it has become). The purity of science has been left in a by-gone era, I'm afraid.
Therefore the onus falls to you to always be vigilant and discerning. I ask you to think. Be informed. Starve for information. Search, always. Maintain healthy skepticism. Always beg to be convinced with sound evidence and empirical data. I often return to my lifelong instinct or gut feeling on things; if it doesn't feel smooth, beauteous and symmetrical in your mind, then there may be something not quite right with it. You have to remember that nature tends to drift to systems of simplicity and efficiency so if a theory put forward by a person or group of persons is getting too convoluted and complicated (probably to justify or patch its increasing bulk) it may be suspect. Don't misunderstand me, complicated isn't at all bad, it just starts to smell a bit fishy if it begins to get circuitous.
Anyway...That's all. I have lots of other things to comment on, but for now...
thanks for playing and I hope your head doesn't hurt too much by now...